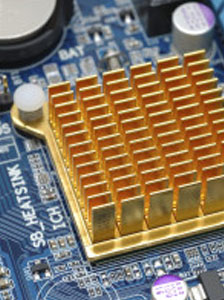
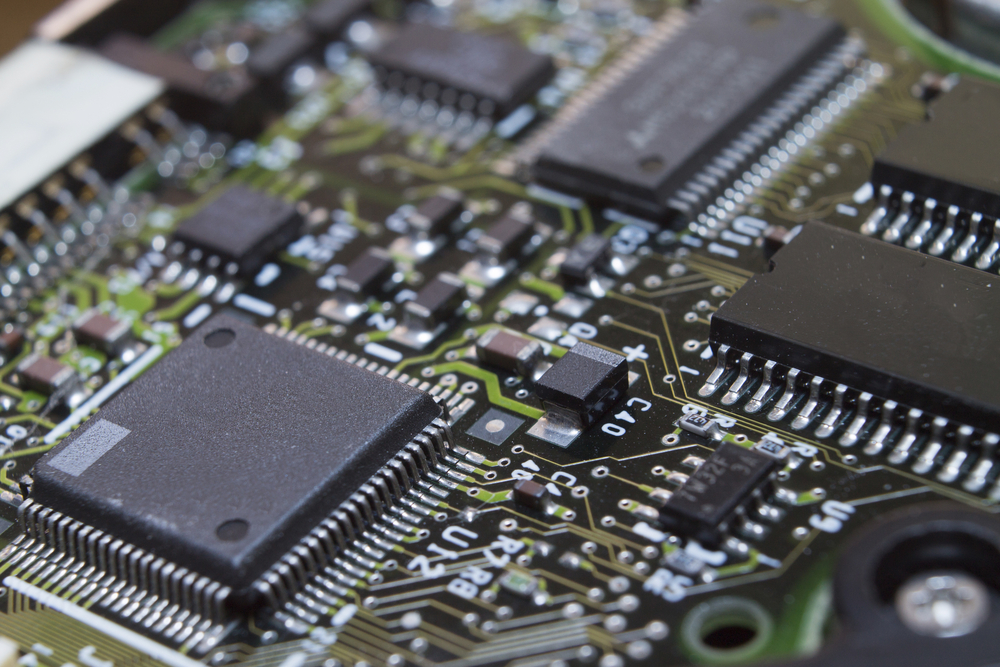
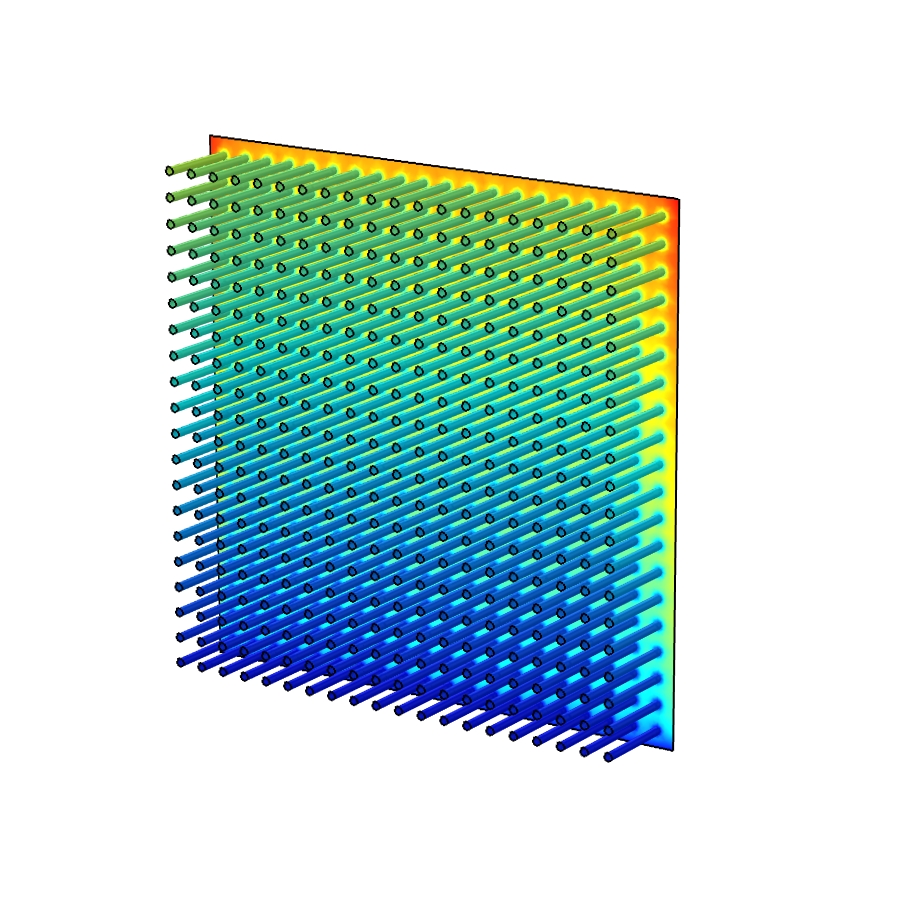
Problem: As Rockwell Automation looked to improve product functionality, engineers relied on developing physical prototypes to test heat sink designs for its powerful microprocessor chips. This approach left mechanical engineers scrambling at the end of the design cycle to find an electronics cooling solution that would fit the prototypes and not delay new product releases. …
HeatSinkSim Changing Electronics Cooling It has been a while since we have put out a Blog on electronics cooling and there is a very good reason for that – not much has changed, until now. Progressive companies manufacturing electronic components and circuits consistently challenge the limits of component performance by offering increased functionality …
Heat Sink Design In last month’s Blog we discussed alternative techniques for increasing the amount of heat dissipation in electronic circuits and components. One of the most commonly used approaches to increase heat dissipation is the use of heat sinks. Heat sink design seeks to maximize the surface area in contact with the surrounding …
Thermal Mitigation – Common Approaches If the operating temperatures remain outside the prescribed thermal budget established for safe device and component operation we are now faced with two options: either reduce the power levels that may change device functionality or increase the dissipation of heat to reduce the standard operating temperatures. In some …
So far we have explored preliminary ways to predict the temperatures in a circuit using simple thermal resistance network calculations. This post addresses improved thermal analysis for electronics cooling. Consistent exposure to high operating temperatures can lead to degradation of material properties and development of thermal stresses that can ultimately lead to device failure. The …
Improved Thermal Analysis for Electronics Cooling Read More »
Previously we have discussed ways to complete a feasibility thermal management analysis. For a given thermal budget, we want to determine whether the junction temperature will meet specifications for reliability and thermal runaway. In electronic systems that rely on air flow for cooling, convection is of paramount importance. Power dissipation due to convection …
In an earlier blog the thermal resistance network shown in Figure 1 was developed. Figure 1: The heat dissipation paths from the die are: to the case of the package, and through the BGA into the PCB. Heat is then dissipated through the skin of the device by convection to the environment. The two dissipation …
During a feasibility analysis for electronics cooling solutions, the objective is to estimate the heat flow using engineering approaches and judgment. By applying a thermal resistance network to the heat flow pathways within the system quantitative estimates for heat flow can be developed as an initial screen for feasibility of component design. As an example …
Thermal Resistance Networks for Electronics Cooling Solutions: Initial Set Up Read More »
Many industries face increased thermal challenges with their electronic products. Customers often want devices with smaller form factors and higher functionality, but these changes create higher thermal fluxes and power density, which in turn require better thermal management to prevent device failure. From the electrical designer’s standpoint, getting traction on thermal problems may be …
Beginning evaluation of a system-level thermal solution-Part 1 Read More »
Thermal Management: A growing concern The demand for compact, multi-functional, high performance electronics has led to a dramatic increase in the power density of devices. In particular, the thermal dissipation per area of chip and per volume of system enclosure has increased dramatically in the last decade. To make matters worse, system-level requirements for small …